4.7 Buckingham-Darcy Law

In 1902, a physicist named Edgar Buckingham was hired by the US Department of Agriculture to work in its newly formed Bureau of Soils [12]. He stayed there for only four years, but his studies during that time led to a conceptual breakthrough that would change the course of soil physics and hydrology. Drawing on earlier work by John Maxwell and Lyman Briggs, Buckingham reasoned that in unsaturated soil the water was attracted to and held by the surfaces of the soil solids by what he called “capillary potential”, i.e. matric potential [13]. He saw that spatial gradients in this potential would act as a force to drive soil water flow and that the resulting flow would be proportional to and in the opposite direction of that gradient. Buckingham also correctly predicted that in unsaturated soil the “capillary conductance”, i.e. the hydraulic conductivity, would be largely dependent on the soil water content. And, Buckingham measured the variation of the equilibrium soil water content with height above a water table in long laboratory soil columns, resulting in the first published soil water retention curves relating water content and “capillary potential”.

Because of Buckingham’s careful experimental work and perceptive theoretical insights, subsequent soil physicists were able to formulate a single equation which describes both saturated and unsaturated water flow in soil and other porous media:

(Eq. 4-5)

where K(θ) indicates that the hydraulic conductivity is a function of the soil volumetric water content and d(ψ_p + ψ_g)/dz indicates the gradient of the total water potential in the z direction. Osmotic potential can also be included with the other potentials if necessary. This equation can be viewed as a generalization of Darcy’s Law and can be referred to as the Buckingham-Darcy Law.

To understand unsaturated water flow in soil, we need to appreciate at least three key differences between Darcy’s Law and the Buckingham-Darcy Law. First, we need to understand that the hydraulic conductivity depends strongly on the soil water content in unsaturated soil and that water content can vary in space and time. For example, the hydraulic conductivity of Grenoble sand is near 15 cm h^-1 when the water content is 0.30 cm³ cm^-3, but the hydraulic conductivity drops more than one order of magnitude, to approximately 1 cm h^-1 when the water content decreases to 0.20 cm³ cm^-3 (Fig. 4‑7, [14]). The cause of this dramatic sensitivity to water content is linked to what we learned previously about the soil water retention curve, capillary rise, and Poiseuille’s Law.

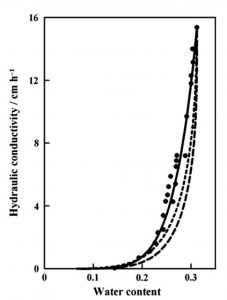
From the soil water retention curve, we know that soil water content is positively related to soil matric potential, so if the soil water content decreases from 0.30 to 0.20 cm³ cm^-3, a corresponding decrease in matric potential must occur. From the capillary rise equation, we can infer that as matric potential decreases (analogous to an increase in capillary rise), water is held in capillary spaces with smaller radii, r. And from Poiseuille’s Law, we know that flow through a capillary depends on r². So if the capillary radius is reduced by a factor of 4, then the flow would be reduced by a factor of 16. Now, soils are not capillary tubes, but this line of reasoning helps us to understand and explain why hydraulic conductivity depends so strongly on soil water content.

The second key difference between Darcy’s Law and the Buckingham-Darcy Law is that, for the latter, the gradient driving the flow depends on the soil water content. The gradient term in the Buckingham-Darcy Law includes ψ_p, the pressure potential. For unsaturated soil the pressure potential term includes the matric potential, ψ_m, which is related to the soil water content by the water retention curve. Thus, within an otherwise homogeneous soil, gradients in water content indicate gradients in matric potential which drive soil water flow from areas of higher water content to areas of lower water content.

A third key difference between Darcy’s Law and the Buckingham-Darcy Law is a consequence of the first two differences highlighted. Darcy’s Law can be applied directly to determine the flux through a layer of saturated soil of any desired thickness, so long as the hydraulic conductivity and the hydraulic gradient are defined for that layer. But, the Buckingham-Darcy Law as written above cannot be applied directly to determine the flux through a layer of unsaturated soil when the water content varies throughout the layer. In those situations, the hydraulic conductivity and the hydraulic gradient can vary by orders of magnitude within the layer, so no single value for those variables can be used to represent the layer. That is why the Buckingham-Darcy law is written as a differential equation with the term d(ψ_p + ψ_g)/dz indicating that the gradient is defined for a layer of infinitesimal thickness. The corresponding hydraulic conductivity must also be evaluated for the water content of that infinitesimal layer, and the product of the gradient and the conductivity gives the flux for that vanishingly thin layer.

To calculate the flux across a real soil layer of finite thickness, the Buckingham-Darcy Law must be integrated across that layer. We will encounter an example of this approach later when we study evaporation from an underground water table. However, such integration is possible only for some relatively simple and specific flow problems. In other cases, it is possible to apply some simplifying assumptions to the Buckingham-Darcy Law, such as assuming a unit hydraulic gradient, resulting in an algebraic expression to calculate the flux at a particular depth in the soil. We will see an example of this approach when we study soil water redistribution and deep drainage.