3.2 Soil Water Potential

Knowing the soil water content is useful for many applications, but there is another variable which is equally important to understanding soil water processes, and that variable is called soil water potential. Soil water potential is a measure of the potential energy of the soil solution. Potential energy is energy that a substance or object has by virtue of its location or internal condition, and potential energy is always defined relative to some reference condition. For example, a book resting on a table has less potential energy than the same book would have if you held it in the air above the table. If you release the book, it will spontaneously fall back down to the table due to the force of gravity. In this case, the increased potential energy came from raising the book upward against the Earth’s gravitational field, and the book’s spontaneous fall when released illustrates the general tendency of things to move from locations of higher to lower potential energy.

3.2.1   Soil Water Potential Terminology

Thus, soil water potential (ψ) is the potential energy of the soil solution relative to that of water in standard reference state. By convention, that state is defined by:

• pure water
• at atmospheric pressure
• at the same temperature as the soil solution
• at a specified, constant elevation.

Differences in soil water potential cause water in soil to flow from regions of higher water potential to regions of lower water potential. When the water potential is equal throughout a certain region of soil, then that region has reached a condition called equilibrium. At equilibrium, there is no water flow. The concept of equilibrium is important for theoretical purposes and laboratory experiments but rarely, if ever, occurs in the field.

Most scientists consider the total soil water potential to be the simple sum of component potentials from several contributing factors, most notably: gravitational potential, osmotic potential, and pressure potential.

• Gravitational potential (ψ_g) is the potential energy which is due to the position of substance or object in a gravitational field. Gravitational potential is measured with respect to an arbitrary reference elevation.
• Osmotic potential (ψ_o) is the decrease in the water potential which is due to the presence of solutes. Osmotic potential affects soil water flow whenever there is a gradient in solute concentration within the soil. Such gradients are especially persistent where there is a membrane, such as the wall of a plant cell, or a barrier, such as the soil surface, that restricts the movement of either water or solutes.
• Pressure potential (ψ_p) refers to the soil water potential attributable to any other factors besides gravity and solutes. Factors such as capillarity, adsorption, submergence, and air pressure can all influence the pressure potential.
  • Matric potential (ψ_m) is an important subcategory of pressure potential. Matric potential is the decrease in the water potential due to the interaction of water with the soil’s solid matrix, primarily through capillarity and adsorption.

Soil water potential can be expressed in a variety of different ways, creating some opportunity for confusion. The three most common ways of expressing soil water potential are:

• Energy per unit mass (J kg^-1) is perhaps the most fundamental and theoretically sound way to express soil water potential but is not as widely used the other two.
• Energy per unit volume (J m^-3 or kPa) has the same units as pressure and is convenient for expressing pressure potential and osmotic potential. However, some uncertainty can arise with this form of expression since the density of water, and thus the relation of mass to volume, is slightly temperature dependent.
• Energy per unit weight (J N^-1 or m or cm) is sometimes referred to as hydraulic head and, when the water flow velocity is negligibly small, is indicated by the height of the free water surface above or below a specified reference elevation. This way of expressing water potential is often convenient for solving soil water flow problems.

      In this book we will primarily use energy per unit volume (kPa) and energy per unit weight (m or cm) to express soil water potential. Soil water potentials vary over several orders of magnitude.  A soil in which the pore spaces are completely filled with water would have a matric potential of 0 kPa, while a surface soil exposed to dry atmospheric conditions for extended periods of time would have a matric potential far below -1500 kPa.

3.2.2   Soil Water Potential Measurement Techniques

Measurements of soil water potential or its components are used by researchers to understand water movement in the soil-plant-atmosphere continuum and by crop and turf managers to guide irrigation management. The oldest type of measuring device for this purpose is called a tensiometer, which consists of a ceramic cup connected to a water reservoir and a pressure gauge (Fig. 3‑3). When the cup is embedded in the soil, water flows into or out of the reservoir through the ceramic until the water potential inside the cup is equal to the water potential outside the cup.

A more recent measurement technique for matric potential utilizes heat dissipation sensors which can provide matric potential measurements at potentials far below the tensiometer range. Heat dissipation sensors consist of a small temperature sensor and a heating element encased in the center of a ceramic plug (Fig. 3‑4). When the sensor is embedded in soil, water moves into or out of the ceramic until the matric potential inside the ceramic equilibrates with the matric potential in the surrounding soil. A pulse of electric current passed through the heater generates a temperature rise which is recorded using the temperature sensor.The movement of water into or out of the reservoir causes the pressure at the location of the pressure gauge to increase or decrease relative to atmospheric pressure. The gauge pressure, corrected for the height of the water column between the cup and the gauge, indicates the pressure potential or matric potential at the location of the cup. Tensiometers do not indicate osmotic potential because solutes can move freely through the ceramic. Tensiometers are typically limited to measuring pressure potentials greater than approximately -80 kPa, below which air passes through the ceramic and invalidates the pressure gauge reading.

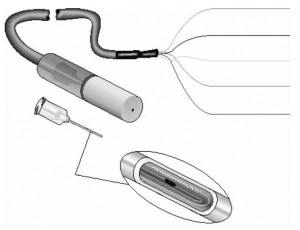
Soil water potential can also be measured on soil samples in the laboratory using an instrument such as a dewpoint potentiometer. A small soil sample is placed inside the instrument in a sealed chamber containing a small mirror (Fig. 3‑5). The water potential of the air in the space above the sample equilibrates with the soil water potential, and the instrument lowers the temperature of the mirror until finding the precise temperature at which dew forms on the mirror. Based on this dewpoint temperature, the water potential of the soil sample is determined. This instrument can measure water potentials over a large range but is even less sensitive in the wet range than are heat dissipation sensors. Current dewpoint potentiometers have an upper limit of approximately -100 kPa.The magnitude of the temperature rise is related to the absolute value of the matric potential. If the soil and ceramic are relatively dry, the temperature rise will be large, and the matric potential will be some large negative number. If the soil and ceramic are relatively wet, the temperature rise will be small, and the matric potential will be some small negative number. Heat dissipation sensors lose sensitivity when the ceramic is saturated and thus have an upper measurement limit of approximately -10 kPa.